From Wikipedia, the free encyclopedia

The Canadarm2 robotic manipulator on the International Space Station is operated by controlling the angles of its joints. Calculating the final position of the astronaut at the end of the arm requires repeated use of trigonometric functions of those angles.
Trigonometry (from Greek trigōnon, "triangle" and metron, "measure"[1]) is a branch of mathematics that studies relationships involving lengths and angles of triangles. The field emerged during the 3rd century BC from applications of geometry to astronomical studies.[2]
The 3rd-century astronomers first noted that the lengths of the sides of a right-angle triangle and the angles between those sides have fixed relationships: that is, if at least the length of one side and the value of one angle is known, then all other angles and lengths can be determined algorithmically.
These calculations soon came to be defined as the trigonometric functions and today are pervasive in both pure and applied mathematics: fundamental methods of analysis such as the Fourier transform, for example, or the wave equation, use trigonometric functions to understand cyclical phenomena across many applications in fields as diverse as physics, mechanical and electrical engineering, music and acoustics, astronomy, ecology, and biology. Trigonometry is also the foundation of surveying.
Trigonometry is most simply associated with planar right-angle triangles (each of which is a two-dimensional triangle with one angle equal to 90 degrees). The applicability to non-right-angle triangles exists, but, since any non-right-angle triangle (on a flat plane) can be bisected to create two right-angle triangles, most problems can be reduced to calculations on right-angle triangles. Thus the majority of applications relate to right-angle triangles. One exception to this is spherical trigonometry, the study of triangles on spheres, surfaces of constant positive curvature, in elliptic geometry (a fundamental part of astronomy and navigation). Trigonometry on surfaces of negative curvature is part of hyperbolic geometry.
Trigonometry basics are often taught in schools, either as a separate course or as a part of a precalculus course.
History

Hipparchus, credited with compiling the first trigonometric table, is known as "the father of trigonometry".[3]
Sumerian astronomers studied angle measure, using a division of circles into 360 degrees.[4] They, and later the Babylonians, studied the ratios of the sides of similar triangles and discovered some properties of these ratios but did not turn that into a systematic method for finding sides and angles of triangles. The ancient Nubians used a similar method.[5]
In the 3rd century BCE, classical Greek mathematicians (such as Euclid and Archimedes) studied the properties of chords and inscribed angles in circles, and they proved theorems that are equivalent to modern trigonometric formulae, although they presented them geometrically rather than algebraically.
The modern sine function was first defined in the Surya Siddhanta, and its properties were further documented by the 5th century (CE) Indian mathematician and astronomer Aryabhata.[6] These Greek and Indian works were translated and expanded by medieval Islamic mathematicians. By the 10th century, Islamic mathematicians were using all six trigonometric functions, had tabulated their values, and were applying them to problems in spherical geometry.[citation needed] At about the same time, Chinese mathematicians developed trigonometry independently, although it was not a major field of study for them. Knowledge of trigonometric functions and methods reached Europe via Latin translations of the works of Persian and Arabic astronomers such as Al Battani and Nasir al-Din al-Tusi.[7] One of the earliest works on trigonometry by a European mathematician is De Triangulis by the 15th century German mathematician Regiomontanus. Trigonometry was still so little known in 16th-century Europe that Nicolaus Copernicus devoted two chapters of De revolutionibus orbium coelestium to explain its basic concepts.
Driven by the demands of navigation and the growing need for accurate maps of large geographic areas, trigonometry grew into a major branch of mathematics.[8] Bartholomaeus Pitiscus was the first to use the word, publishing his Trigonometria in 1595.[9] Gemma Frisius described for the first time the method of triangulation still used today in surveying. It was Leonhard Euler who fully incorporated complex numbers into trigonometry. The works of James Gregory in the 17th century and Colin Maclaurin in the 18th century were influential in the development of trigonometric series.[10] Also in the 18th century, Brook Taylor defined the general Taylor series.[11]
Overview

If one angle of a triangle is 90 degrees and one of the other angles is known, the third is thereby fixed, because the three angles of any triangle add up to 180 degrees. The two acute angles therefore add up to 90 degrees: they are complementary angles. The shape of a triangle is completely determined, except for similarity, by the angles. Once the angles are known, the ratios of the sides are determined, regardless of the overall size of the triangle. If the length of one of the sides is known, the other two are determined. These ratios are given by the following trigonometric functions of the known angle A, where a, b and c refer to the lengths of the sides in the accompanying figure:
- Sine function (sin), defined as the ratio of the side opposite the angle to the hypotenuse.
-
sinA=oppositehypotenuse
-
cosA=adjacenthypotenuse.
- Tangent function (tan), defined as the ratio of the opposite leg to the adjacent leg.
-
tanA=oppositeadjacent=ac∗cb=ac/bc=sinAcosA.
The hypotenuse is the side opposite to the 90 degree angle in a right triangle; it is the longest side of the triangle and one of the two sides adjacent to angle A. The adjacent leg is the other side that is adjacent to angle A. The opposite side is the side that is opposite to angle A. The terms perpendicular and base are sometimes used for the opposite and adjacent sides respectively. Many people find it easy to remember what sides of the right triangle are equal to sine, cosine, or tangent, by memorizing the word SOH-CAH-TOA (see below under Mnemonics).
The reciprocals of these functions are named the cosecant (csc or cosec), secant (sec), and cotangent (cot), respectively:
cosecA=1sinA=hypotenuseopposite=ca,
secA=1cosA=hypotenuseadjacent=cb,
cotA=1tanA=adjacentopposite=cosAsinA=ba.
With these functions one can answer virtually all questions about arbitrary triangles by using the law of sines and the law of cosines. These laws can be used to compute the remaining angles and sides of any triangle as soon as two sides and their included angle or two angles and a side or three sides are known. These laws are useful in all branches of geometry, since every polygon may be described as a finite combination of triangles.
Extending the definitions
The above definitions only apply to angles between 0 and 90 degrees (0 and π/2 radians). Using the unit circle, one can extend them to all positive and negative arguments (see trigonometric function). The trigonometric functions are periodic, with a period of 360 degrees or 2π radians. That means their values repeat at those intervals. The tangent and cotangent functions also have a shorter period, of 180 degrees or π radians.
The trigonometric functions can be defined in other ways besides the geometrical definitions above, using tools from calculus and infinite series. With these definitions the trigonometric functions can be defined for complex numbers. The complex exponential function is particularly useful.
ex+iy=ex(cosy+isiny).
- Graphing process of y = sin(x) using a unit circle.
Mnemonics
A common use of mnemonics is to remember facts and relationships in trigonometry. For example, the sine, cosine, and tangent ratios in a right triangle can be remembered by representing them and their corresponding sides as strings of letters. For instance, a mnemonic is SOH-CAH-TOA:[12]- Sine = Opposite ÷ Hypotenuse
- Cosine = Adjacent ÷ Hypotenuse
- Tangent = Opposite ÷ Adjacent
Calculating trigonometric functions
Trigonometric functions were among the earliest uses for mathematical tables. Such tables were incorporated into mathematics textbooks and students were taught to look up values and how to interpolate between the values listed to get higher accuracy. Slide rules had special scales for trigonometric functions.Today scientific calculators have buttons for calculating the main trigonometric functions (sin, cos, tan, and sometimes cis and their inverses). Most allow a choice of angle measurement methods: degrees, radians, and sometimes gradians. Most computer programming languages provide function libraries that include the trigonometric functions. The floating point unit hardware incorporated into the microprocessor chips used in most personal computers has built-in instructions for calculating trigonometric functions.[14]
Applications of trigonometry

Sextants are used to measure the angle of the sun or stars with respect to the horizon. Using trigonometry and a marine chronometer, the position of the ship can be determined from such measurements.
Fields that use trigonometry or trigonometric functions include astronomy (especially for locating apparent positions of celestial objects, in which spherical trigonometry is essential) and hence navigation (on the oceans, in aircraft, and in space), music theory, audio synthesis, acoustics, optics, electronics, probability theory, statistics, biology, medical imaging (CAT scans and ultrasound), pharmacy, chemistry, number theory (and hence cryptology), seismology, meteorology, oceanography, many physical sciences, land surveying and geodesy, architecture, image compression, phonetics, economics, electrical engineering, mechanical engineering, civil engineering, computer graphics, cartography, crystallography and game development.
Pythagorean identities
Identities are those equations that hold true for any value.sin2A+cos2A=1
sec2A−tan2A=1
csc2A−cot2A=1
Angle transformation formulae
sin(A±B)=sinA cosB±cosA sinB
cos(A±B)=cosA cosB∓sinA sinB
tan(A±B)=tanA±tanB1∓tanA tanB
cot(A±B)=cotA cotB∓1cotB±cotA
Common formulae
Certain equations involving trigonometric functions are true for all angles and are known as trigonometric identities. Some identities equate an expression to a different expression involving the same angles. These are listed in List of trigonometric identities. Triangle identities that relate the sides and angles of a given triangle are listed below.
In the following identities, A, B and C are the angles of a triangle and a, b and c are the lengths of sides of the triangle opposite the respective angles (as shown in the diagram).
Law of sines
The law of sines (also known as the "sine rule") for an arbitrary triangle states:asinA=bsinB=csinC=2R=abc2Δ,
R=abc(a+b+c)(a−b+c)(a+b−c)(b+c−a)−−−−−−−−−−−−−−−−−−−−−−−−−−−−−−−−−√.
Area=Δ=12absinC.
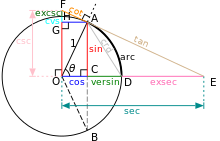
All of the trigonometric functions of an angle θ can be constructed geometrically in terms of a unit circle centered at O.
Law of cosines
The law of cosines (known as the cosine formula, or the "cos rule") is an extension of the Pythagorean theorem to arbitrary triangles:c2=a2+b2−2abcosC,
cosC=a2+b2−c22ab.
s=12(a+b+c),
Area=Δ=s(s−a)(s−b)(s−c)−−−−−−−−−−−−−−−−−√=abc4R ,
Law of tangents
The law of tangents:a−ba+b=tan[12(A−B)]tan[12(A+B)]
Euler's formula
Euler's formula, which states thatsinx=eix−e−ix2i,cosx=eix+e−ix2,tanx=i(e−ix−eix)eix+e−ix.